Death Comes (and Comes and Comes) to the Quantum Physicist

I. A Resurrection of Cats

In 1957, a physics graduate student at Princeton, Hugh Everett III, proposed a ­cryptic and seductive solution to the central paradox of quantum mechanics. He argued that the paradox—roughly stated, that the Schrödinger equation perfectly predicts an electron’s behavior in every quantum mechanical experiment ever imagined but also entails some sort of nonsense about an unobserved cat being simultaneously alive and dead—is in fact no paradox at all. Everett made a case for reading the Schrödinger equation literally. The cat is both dead and alive: dead in one world, and still alive in another. One consequence of Everett’s idea is that the universe consists of infinite worlds, embodying every possibility, such that, for example, in one world I die of rheumatic fever in childhood, in another I’m writing a slightly different version of this article, in most I do not exist at all, and, in a few, you and I are in love. Everett’s argument, originally set out in the rather prosaically titled “The Theory of the Universal Wave Function,” re­ceived virtually no attention, after which the dispirited young Everett left academia forever. Applying his mathematical innovations to private and government de­fense analysis projects, he became a heavy-drinking, chain-smoking millionaire; his em­ployees say he avoided mention of his physics background altogether.

Thirteen years later, in 1970, the physicist Bryce DeWitt published in Physics Today a short ­article, “Quantum Mechanics and Reality.” DeWitt, one of the few academics who had noticed Ever­ett’s work, began:

Despite its enormous practical success, quantum theory is so contrary to intuition that, even after 45 years, the experts themselves still do not all agree what to make of it. The area of disagreement centers primarily around the problem of describing observations… Of the three main proposals for solving this di­lemma, I shall focus on one that pictures the universe as continually splitting into a multiplicity of mu­tually unobservable but equal­ly real worlds… Although this proposal leads to a bizarre world view, it may be the most satisfying answer advanced yet.

DeWitt’s flatly sincere, hand-holding article, which led to the catchy “Many Worlds” moniker for Everett’s long-ignored idea, elicited an enormous response from the physics community. Physics Today ran a follow-up article half a year later, with six (handsomely photograph­ed) physicists responding at some length to DeWitt, and DeWitt responding more in kind. Many Worlds was thus officially sexy, and by 1973 Everett’s dormant thesis work was finally, through DeWitt’s arrangement, published. Seemingly aloof to the renewed interest in his hypothesis, Everett claimed he couldn’t be bothered to write an introduction. So DeWitt wrote one instead. As epigraphs he used quotes from Borges and William James.

Soon thereafter the elder statesman of science fiction magazines, ANALOG (formerly known as Astounding Stories!), featured, in its “Science Fact” column, a lengthy discussion of the Many Worlds Interpretation (MWI). The subheading: “Alternate universes are not merely gimmicks for SF ­writers—they’re necessary for the salvation of quantum physicists!” Again, interest, if of a slightly different flavor, was enormous. Ev­erett—by then spending his time on classified consulting projects such as the development of a Quick General War Game Simulator for the De­partment of De­fense—was rapidly becoming a rock star in the science and science fiction communities both. When he made his one and only post-fame appearance at an academic conference in Austin in 1977, ­student groupies engulfed him, the no-smoking rules for the auditorium were suspended exclusively for the duration of his four-hour talk, and internet debate continues to this day concerning the rumor that Everett arrived at the conference in a black Cadillac with horns.

But just what Everett’s pro­posal actually means—or should have meant—remains ambiguous; Ever­ett was never successfully lured back to academia, and, in 1982, at age fifty-one, he died of a heart attack. (Or, if one is to believe rumors on the internet, he died at the hands—or claws?—of aliens angered by the global-scale UFO research Everett conducted on be­half of the Pentagon; recent declassification of Pentagon documents cor­roborates the existence of the re­search but not the aliens.) De­spite the semi-embittered withdrawal of its originator, the core of Everett’s idea has aged quite well. Its earliest flaws have been (ar­guably) remedied, and among the several interpretations of quantum mechanics currently under serious consideration, many prominent physicists—Stephen Hawking, Murray Gell-Mann—subscribe to MWI, holding not simply that it’s a successful method for making scientifically accurate predictions but  also that it’s literally true. In fact, Ox­ford’s most revered recluse, the physicist David Deutsch, claims Everett’s Many Worlds Interpretation of quantum mechanics as one of four strands of a Theory of Everything. (The other three strands, if you’re curious, are Ri­chard Dawkins’s refinements of Dar­winian evolution, Alan Turing’s theory of computation, and Karl Popper’s epistemology.) However, others’ as­sessments of Everett’s idea are closer in spirit to that of the brilliant Irish physicist J. S. Bell, who wrote in 1981 that “if such a theory were taken seriously it would hardly be possible to take any­thing else seriously.”

This year, with Everett’s thesis work turning fifty, several conferences are dedicated to forcing the question of whether the Many Worlds Interpretation might be, regardless of its alluring, nightmarish beauty, actually true. That the Everett interpretation is “too fantastic” is, according to the philosopher of science and MWI proponent Simon Saunders, “not the ob­jection that is usually made… however much it may weigh privately.” Instead the debate focuses on how Everett’s hypothesis ex­plains (or doesn’t) quantum probabilities. This leads (eventually) to questions like: Is personal identity conserved across parallel­iverses? Does the Everett worldview entail a tortured immortality, the scien­tific equivalent of a living hell?

II. The Problem of Knife-wielding Descendants

Long before they were discussed in scholarly scientific articles, alternate parallel universes appeared in science fiction; they offered a fresh solution to what is known as the grandfather paradox, a problem articulated early on in a seventeen-year-old’s letter to the editor printed in the December 1931 issue of Astounding Stories!:

There is only one kind of Science Fiction story that I dislike, and that is the so-called time-traveling… supposing a man had a grudge against his grandfather… He could hop in his machine and go back to the year that his grandfather was a young man and murder him. And if he did this how could the revenger be born? I think the whole thing is the “bunk.”

Die-hard science fiction readers, it turns out, are often the most radical devotees of realism. (Even the revered H. G. Wells received critical scolding for not addressing the grandfather paradox in his genre-generating 1895 The Time Ma­chine.) One old narrative solution to the grandfather paradox simply stipulates that something—damp dynamite, a shoddy pistol, oxidized poison, Hamlettian indecision, ba­nana peels, heroic bystanders, an epi­leptic fit, anything really—in­evitably foils even the best-laid of homicidal time-traveling plans. However, for science fiction realists, this solution fails to explain why such snafus are unavoidable.

A second oft-used technique de­ploys some variation of time cops: brave, just forces upholding the status quo of all eternity. But the revered late philosopher of coun­ter­factuals David Lewis termed this kind of solution to the grandfather paradox “a boring invasion.” Boring in the sense that it makes no contribution to philosophical considerations of the problems of time travel, since no one thinks these officers for the maintenance of history might ­really exist. They just make for a nice story.

But (even before Everett) science fiction writers invented alternate parallel universes as a third way out of the grandfather paradox. Although these paralleliverses existed already in Hindu cosmo­logy, and various writers had feat­ured one parallel world that served as a sort of Heaven (C. S. Lewis) or Hell (H. P. Lovecraft), it was really the grandfather paradox that led to the forking paths of time seen in most science fiction. The cohering idea being that if the traveled-to past belongs not to the murdering grandchild’s original world but in­stead to a parallel alternate one—if that is the only past he can travel to, if that in fact is the way that time travel works—then the grandfather paradox politely disappears. Other merely but usefully emotional problems arise instead—the un­canny loneliness, say, of being stranded in a slightly alternate fork of time.

(For the curious realists among us: Recent physics research into a mathematically simplified version of the grandfather paradox—a billiard ball that enters a wormhole, from which it emerges into the past, where it may knock its former self out of the path it originally traveled to enter the wormhole in the first place—lends the most credence to the goofy first proposal. Apparently grandfather paradoxes are avoided simply by obeying the known laws of physics; it’s analogous to not being able to square a circle. But this discovery surprised its researchers, and its odd upshot is that, in a scenario with backward time travel, possible but extraordinarily unlikely scenarios—the phy­sics equivalents of slipping on a ba­nana peel at precisely the wrong moment—suddenly become the norm.)

The parallel worlds solution to time travel paradoxes is put into fictional play as early as 1938, in sci-fi godfather Jack Williamson’s novel The Legion of Time. Parallel worlds time travel also appears in some of Philip K. Dick’s early stories from the 1950s. And, just a few years before Everett’s thesis, Ward Moore published the acclaimed Bring the Jubilee, which featured a historian stranded in an alternate world’s version of the American Civil War. In general the physics of these fictions doesn’t quite work out; but even in physics the physics often doesn’t quite work out. For example: the conundrum of the Schrödinger equation.

So one small cheer for the in­ventiveness of fiction writers might be appropriate. These stories did predate Everett, after (or before) all. However, although Everett was certainly aware of the science fiction community (his close friend from college was the future ­science fiction writer Karen Kruse, who later married and coauthored books with the Time Patrol sci-fi series writer Poul Anderson), to look at these early fiction works as precursors to Everett, or influences on him, would be to make the error of associating on the most superficial level. Everett wasn’t trying to justify time travel. And he didn’t start out with the idea of parallel worlds; he was just unusually willing to accept the possibility of them in the course of other considerations.

III. Must Metaphysicians Seek to Astound?

Although to the layperson, and even the not-so-layperson, MWI’s initial al­lure perhaps lies in its extravagant oddness—or in its Conradian realization of those other lives we might have lived, or in its Sebaldian returning to us of all things lost, or in its Ecoish assurance that the world may outdo our wildest imag­inings of it—a more sophisticated understanding of Everett’s idea reveals its other charms. MWI can be understood as an exceedingly reasonable attempt to cure quantum mechanics of its grossest ec­centricities, as well as a discreetly im­passioned argument for restoring to physics not only its declining claims to realism but its declining claims to being the philosophically sought-after “view from no­where”—the God’s-eye view. To begin to understand this other side of Everett’s idea—to see the sort of flatfooted, unromantic ra­tionality of MWI—necessitates some un­der­standing of quantum me­chanics, particularly around its most confounding point, what has come to be known as the Measurement Problem.

The Measurement Problem proves itself to be a kind of a postmodern detective story, in which the detective discovers his own role in the investigated crime.

Quantum mechanics—which arose in the 1920s in large part as a re­sponse to the straightforward mystery of why negatively charged electrons don’t crash into their positively charged nuclei—describes the physical laws governing the mo­vement of the smallest stuff of the universe. And since all big stuff is just a crowd of little stuff, quantum mechanics is, actually, if it’s the physics of anything, the physics of everything. This physics has proved itself to be counterintuitive and strange. In fact, so much so that the Nazi physicists Philipp Lenard and Johannes Stark, both brilliant Nobel laureates, dismissed early quantum mechanics (along with Ein­­stein’s relativity) as degenerate Jewish science, full of spurious ideas “unencumbered by regard for truth.” The confusion, if not the re­sulting conclusion, continues to the present day. As Richard Feynman famously remarked, “I think I can safely say that no one understands quantum mechanics.”

Superposition, nonlocality, and randomness are the central strangenesses of quantum mechanics. Su­per­position refers to the fact that electrons manage to be, in some very real sense, in two noncompatible states at once, which means an atom manages to be both decayed and not decayed, and consequently a cat both alive and not. Non­locality entails that particles un­fathomable distances apart can have direct and instantaneous effects on each other; one can imagine a fist thrown in one corner of the universe leading, immediately and without intermediaries, to the breaking of a nose in a galaxy far, far away. And weirdness number three, randomness, means that, contrary to what scientists were led to be­lieve by three hundred stun­ningly successful years of Newton’s de­terministic mechanics, the fundamental laws of the universe in­volve pure chance. God, as they say, ap­parently does play dice.

The Measurement Problem ir­ritated young Everett enough to make his dubious pearl. If Everett’s hy­pothesis is correct—and this is what excites the scientists—the Measurement Problem simply disappears, as if it never was.

Here’s one example of the Measure­ment Problem in its characteristically aloof mysteriousness:

Electrons have a measurable property called x-spin, which can be either |up> or |down>; it doesn’t really matter what this property is or does, we could just as easily call it jealousy and be asking whether the electron is or isn’t ­jealous. But better to stick with the misleading appeal of the dry language. The principle of superposition stipulates that, for any two physically possible states of any physical system (the possible states |up> and |down> of the electron just mentioned, for example), a “superposition” of those two states, which we can mathematically represent as 1/√2 |up> + 1/√2 |down>, is physically possible as well.

The way the quantum state of a system varies over time is supposed to be governed by the Schrödinger equation, which reads:

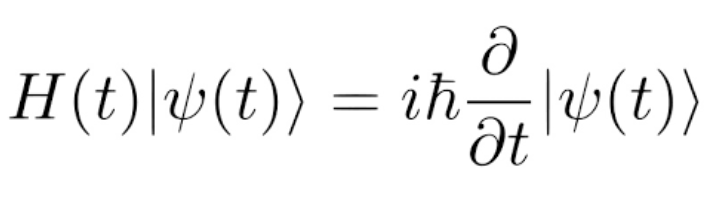
Which is (not wholly irrelevantly) kind of pretty; and which describes the electron’s x-spin as that strange thing, a superposition, a sum of part x-spin up and part x-spin down. Yet whenever anyone has actually looked (however sneakily) at an electron’s x-spin, it has been found to be either up or down. Never both and never something somehow in between. The Schrödinger equation itself im­plies the superposition, but Schrödinger himself pointed out the preposterousness of this. He made more vivid the unintellig­ibility of his equation by magnifying it in his famous cat paradox: if one sets up an experiment such that an x-spin being “up” leads (via the Schrö­dinger equation) to the re­lease of a deadly poisonous gas into a box in which a kitty is kept, and an x-spin being “down” does not lead (via the Schrödinger equation) to the poisonous gas release, then, when the spin is in a superposition, the cat is, in some sense (though no one knows quite what sense), in a superposition of being poisoned and not poisoned, of being dead and alive. But we’ve never seen a cat like that. So what could that even mean? It would seem the equation is simply wrong.

The rub is that, despite this od­dity, the Schrödinger equation has perfectly predicted the way wave functions evolve whenever we’re not making measurements, when we’re not opening the box to see if the cat is alive or dead. And when you combine the Schrödinger equation with a simple rule about what happens when you do make measure­ments (the measured system randomly settles into one component of the superposition), you get perfectly accurate predictions of the outcome of absolutely any experiment. That’s why quantum mechanics has been accepted for about eighty years now as the physics of our universe, as more fundamentally true, even, than F=ma (which is, by comparison, a mere shadowy approximation). Only in the isolated cases of making measurements, of checking on the cat, does something seem amiss, or nonsensical. In between measurements it’s perfect. So Schrö­dinger’s equation works flawlessly and yet leads to a logical paradox—a cat both alive and dead?—that we don’t know how to untangle. Which is why the physicist J. S. Bell said of Schrödinger’s equation that it essentially both must be and can’t possibly be true.

So the measurement problem is weird, and Everett’s attempt to make sense of it—the equation spans more than one world, and in one world the cat is dead, in ­another still alive—is by no means the weirdest; it’s arguably one of the most straightforward. Moreover, Everett’s attempt offers the additional “benefits” of unveiling quantum mechanics as actually non­random and local; it does this while si­multaneously explaining why it ap­pears to be random and nonlocal. One might consider this resolution of quantum mechanics’ strange­nesses a point in Everett’s favor.

Many other efforts to understand quantum mechanics’ quirks have been made, and if you want really bizarre, consider Nobel Prize–winning Hungarian physicist E. P. Wigner’s serious speculations that it was consciousness itself that led an indeterminate result (the cat is alive and dead) to randomly be­come, upon measurement—upon lifting the lid of the cat’s box and looking—determinate (the cat is alive or dead). Wigner’s 1962 idea  (which held sway well into the 1980s, with graduate students in experiments trying to “think” electron spins into position) was that the Schrödinger equation is precisely true when we’re not looking, and then, once an observation reaches consciousness, consciousness itself triggers the random “collapse” of the wave function into one or the other terms of the superposition. So under Wigner’s hypothesis, before the emergence of life on earth, there were none of these “col­lapses”; now apparently they’re hap­pening all the time. How often? Wigner once publicly speculated that a dog’s observation likely would cause collapse of the wave function, while a mouse’s most likely would not. Wigner’s consciousness-collapse hypothesis (which, it seems worth noticing, placed humanity at the center of the physical laws of the universe) was taken very seriously; in fact, it was the subject of a highly re­spectable seminar in Austin, Texas, that Everett attended; Everett spoke against the idea.

IV. Against Collapse Interpretations

Wigner’s collapse hy­pothesis belongs to an otherwise venerable and still-thriving tradition of collapse hypotheses, the most famous of which was the von ­Neumann–Dirac collapse formulation—a formulation that an Italian team of physicists led by Giancarlo Ghi­rardi has transformed from an incoherent speculation into a serious scientific theory that today happens to be one of the leading countercontenders to the Many Worlds Interpretation. But in the ’50s, when Everett was working on his thesis, the von Neumann-Dirac col­lapse formulation was the most ac­cepted interpretation of quantum mechanics. It functioned exceptionally well for the “shut up and calculate” approach to physics es­poused by one of its authors, Paul Di­rac. However, its inarguable limitations are what Everett formed his idea against.

The collapse formulation ne­cessitated that observers be treated as external to the system described by the theory; thus the theory could not be considered a complete de­scription of the universe as a whole, since the universe contains ob­servers. Also, the collapse formulation offers a deterministic, continuous, linear rule that holds when a system is not observed, and a random, discontinuous, nonlinear rule when a system is observed. But there’s no precise way to describe or account for just what an observation or a measurement is, and consequently there’s no mathematically precise way of knowing when to apply which rule. A raggedy theory no doubt. Everett wanted to “consider the problem of observation itself within the framework of the theory,” so that physics could have a theory that accounted for everything, that could be complete. A synonym, in science, for beautiful.

In order to accommodate his goal of a complete and universally applicable quantum mechanics, Ever­ett goes on to develop the following interpretation of the seeming superposition described by the Schrödinger equation:

we shall deduce the probabilistic assertions of Process 1 [the random, discontinuous evolution of an observed system] as subjective appearances to such ob­servers, thus placing the theory in correspondence with experience. We are then led to the novel situation in which the formal ­theory is objectively continuous and causal, while subjectively discontinuous and probabilistic… Thus, each element of the resulting superposition describes an ob­server who perceived a definite and generally different result, and to whom it appears that the object-system state has been transformed into the corresponding eigenstate…

So Everett accounts, in his ­theory, for why other theories ap­pear to us to be true. He does this by proposing a theory that agrees with the predictions of a collapse dy­namics, but tries to rescue collapse dynamics from its internal in­consistencies by offering a different reality to which the mathematical results correspond. A primary element of how he achieves this is by making subjective—therefore de­limited, since the observer can only see her world—the measurement an observer believes herself to have observed.

A few intellectual trends contemporary to Everett might be seen as parallels, both in the inclusion in the theory of the ­previously ex­cluded observers, and in the prob­lematizing of the epistemological privileges of the aforementioned included observer. There are hints of structuralist analysis, of psychoanalytic theory. One can also see a connection to the interests of a very young Everett, as evidenced in a letter he sent as a twelve-year-old to Albert Einstein. Everett asked whether something random or unifying held the universe together. Einstein answered: “Dear Hugh: There is no such thing as an irresistible force and im­movable body. But there seems to be a very stubborn boy who has forced his way victoriously through strange difficulties created by himself for this purpose.”

V. When Conservative Radicals Were the Rage

Everett’s advisor at Princeton was the theoretical phy­sicist John Wheeler, and Wheeler’s advisor had been the Danish physicist Niels Bohr. Bohr was the central figure of quantum mechanics and, at the time of Everett’s thesis, arguably the most venerated living physicist in the world. Wheeler sent Everett to Copenhagen for six weeks and ar­ranged for him to speak with Niels Bohr, and the two, indeed, met. Bohr was socially polite, chatty even, but he basically refused to discuss Everett’s work at all. But, perhaps counterintuitively, the larger context reveals Bohr, with his extreme aversion to MWI, as the intellectual (if dogmatic) radical, and Everett, with his nearly in­finite parallel worlds, as the old-fashioned conservative.

Bohr had, in the 1930s, re­sponded to the strangeness of the measurement problem in a way that was briefly heterodoxy, but which then quickly became the new orthodoxy. He declared, basically: that’s what you get for taking physics too seriously, for believing that it gives us a picture of what’s really out there. Bohr’s longtime assistant, the physicist Aage Peterson, paraphrased Bohr’s response this way:

There is no quantum world. There is only an abstract physical description. It is wrong to think that the task of physics is to find out how Nature is. Physics concerns what we can say about Nature.

Bohr argued that for any physics experiment, one must first es­tablish a subject/object split, one must decide on an observer and an ob­served. Following this line of thinking, attending to the requirement of an observer outside of the observed system, Bohr proceeds to the conclusion that physics is there­­fore always relative, and (be­cause the observer cannot be in­cluded) always incomplete. So one might say he had the same concerns as Everett, but resolved them in an entirely other way. According to Bohr, physics fails (everything fails) to achieve the view from no­where; every possible view is perspectival, and physics’ equations can only tell us how certain measurements, made in a certain way, turn out; the real world, as in the tradition of Berkeley, remains ever out of reach. “Measurement,” he writes, “has an essential influence on the conditions on which the very definition of the physical quantities in question rests.” Quantum mechanics’ quirks (the random­ness, the superposition, the non­­locality) in a view like Bohr’s are the empirical cold fish slapping us across the face for ever having en­tertained a realist attitude to­ward mathematics in the first place. E=mc2 doesn’t correspond to the actual real world. We may as well expect to hit our spade against the earth’s equator.

Bohr of course wasn’t the first to express skepticism about how epis­temically available to us the world is, or even how much the world is “there” at all. But the fact that Bohr showed how science itself of­fered an argument against realism—that was startlingly radical. Like watching a prophet disprove his own God. Science, after all, seems predicated on there being a real world out there that we have access to, a world whose laws we can discover through observation. (That’s why a sci-fi reader can be the most dogmatic literary realist.)

Later, in the 1960s, the then radical Maoist philosopher Hilary Put­nam (now a recently bar mitzvah­ed conservative Jew) argued—extending and twisting Bohr’s insight—that what quantum mechanics offered was the proof necessary to overthrow the laws of logic itself. Putnam’s argument stres­sed the fact that quantum mechanics showed us that P and not P can both be true; he then pushed this idea as far as it would go, made it the empirical evidence against logic, something that had perhaps seemed to be apart from empiricism altogether. Later this idea was dismissed (not just by others but also by Putnam), but it shows how powerful arguably wishful (but still incredibly intelligent) the projections placed onto quantum mechanics were (and inevitably still are). After all, can it be pure chance that a radical found in quantum mechanics further support for his radicalism?

Bohr’s radical take on the measurement problem reigned sup­reme for decades. But not over Everett. Because the very premise Everett was working from was to see what happens when we take the Schrö­dinger equation literally, as a de­scription of the actual world. As DeWitt, in his advocacy of Everett’s idea, explained, “Instead of tinkering with the formalism, why not ask what the formalism really says? Why not push it to its logical conclusion?” The Schrö­dinger equation works, after all, so why not “be­lieve” in it? Such a view marked Everett as a scientific realist, someone with the quaint idea that formalism corresponded to reality. Everett’s Many Worlds In­ter­pretation was really a way of saying that Schrödinger’s equation requires no interpretation at all: it means just what it looks like it means, that the cat is both dead and alive. Just in two quantumly entangled but mutually inaccessible worlds. (The physicist Sidney Cole­man liked to call Everett’s ­theory “quantum mechanics in your face.”) Everett’s stance meant foresaking the awesome unintelligibility of the world as read by Bohr. Likely it ­wasn’t Everett’s on­tological ex­travagance that repelled Bohr but his schoolboyish reverence for phy­sics’ equations.

VI. What would Everett do?

Today, if you fill up a room with both detractors and proponents of MWI, they all agree that Bohr dismissed the realist project prematurely; they’re all realists, in search of a realist foundation of physics, which they be­lieve is out there to be found. In a January conference held at Rutgers, the first of three conferences this year addressing ­MWI—another is to be held at Oxford this July, and a third at the Perimeter Institute for Theoretical Physics in September—the discussion centered on whether Everett’s theory can or can’t compellingly account for quantum probabilities.

Consider: If in an Everettian worldview everything quantum mechanically possible does actually happen—the cat gets poisoned and dies, a drummer spontaneously com­busts, the cat miraculously survives the poison, you’re dealt a royal flush, the poor cat scratches you and you become one of the rare fatalities of cat-scratch disease—then why are there reliable probabilities that any given thing will happen? Why do you know so precisely how likely, say, your being dealt a royal flush actually is? This problem—the disparity between what an Everettian would predict and what quantum mechanics actually predicts—is particularly acute be­cause the point at which quantum mechanics intersects our experience is precisely in predicting the probabilities of certain kinds of events, like radioactive decays, or the outcomes of spin measurements, or the viability of cats under various conditions. And quantum me­chanics’ reliable predictions are the only visible part of quantum mechanics, the only reason we have for believing quantum mechanics is true. As MWI skeptic and philosopher Sidney Felder puts it, “Given that the greater observed frequency of outcomes with larger co­efficients provided the initial justification for the whole development of [quantum mechanics]… the Everett picture has placed us in a rather peculiar situation… the Everett in­terpretation undermines the reason for being of the very for­malism whose significance it purports to make transparent.” Why? Because, again, in a Many Worlds multiverse there are no facts about probabilities. Every possibility is realized and therefore the chance of any given possible event happening is 100 percent. And un­like the simple case of the spin measure­ment, where there are just the two possibilities of up and down, most situations involve near-infinite possibilities, like a boulder that may fall down onto picnickers now, ten seconds from now, ten years from now, a millennium from now. Nevertheless, quantum me­chanics itself tells us precisely how much more likely some events are than others—and it has been spectacularly successful in doing so, and that spectacular success is the whole motivation for trying to re­solve its foundational paradox—so how can MWI be a true explanation of quantum me­chanics and yet seem at first blush to generate different probabilities? What does it mean that, in any given second, quantum mechanics can reliably say there’s, for example, a 0.01 percent chance that a certain atom will spontaneously decay, while MWI tells us there’s a 100 percent chance of the same event?

One response might be that the branching isn’t into two worlds (one for each branch of the superposition) but into—in the case of a 0.01 percent probability—ten thousand. And in only one of those ten thousand worlds does the atom decay. So there’s a 0.01 percent chance that “you” end up in the one branch where the decay happened. But, ac­cording to all involved, detractors and supporters of MWI both, such an idea—besides making for an even more ungainly form of in­finity—failed. (The trouble here is that the whole business of counting worlds somehow implicitly takes it for granted that there is a single, real, original you who ends up in some one or another of the final worlds. But that’s not at all what MWI is telling us. In MWI, we end up, for sure, in them all!) An alternate response Ev­erettians have offered to the probability problem is simply to justly make the accusation to MWI skeptics that nobody, with any theory, has ever made sense of probability. This ac­cusation happens to be, most agree, true—just one more example of something we take as a given being frustratingly sucked into a whorl of confusion by a roomful of philosophers one feels inclined to believe are being deliberately exasperating—but in the Rutgers debate, MWI skeptic and philosopher of science David Al­bert countered that “just because no one knows exactly what probability is, doesn’t mean we can say that it’s a kangaroo.”

The Everettians, however, in order to lend credence to their hy­pothesis, have in recent years suggested another rather ingenious way of getting at probabilities. De­cision theory—how a reasonable person would bet in a given situation—stands at the intersection of probabilities and human experience. So if Everettians can provide a compelling account of why a rational agent with a MWI worldview would bet on, say, an atom’s decay in a way that maintains the sense of the statistics of quantum me­chanics, then the probability ob­jection is, inasmuch as it ever is—even if we still can’t say just what probability actually is—solved.

So why wouldn’t an Everettian bet as if he expected to be dealt a royal flush? If the Everettian “ex­pects” to be the average of all his various future selves, the probabilities don’t work out, don’t line up with the predictions of quantum mechanics. But if an Everettian only “expects” to be one of those future selves, the math still doesn’t quite work out. So a further idea has been added, that “in some sense some worlds are larger than others.” The philosopher of science Hilary Greaves calls this a “caring measure.” Others have in­voked an “intensity rule.” The im­plications are similar. We somehow “care more” for the version of ourselves in worlds that quantum me­chanics says are more probable, even though probability here has been—since everything possible in MWI is
100 percent probable—thoroughly problematized. The caring notion isn’t quite as weak as it sounds, but it’s not overwhelmingly compelling either. Detractors ask whether a rational agent should care more about his fat future selves than about his thin future selves because there’s “more of him there.” And questions like whether Ulysses should have tied himself to his mast to sail past the Sirens poke at the problems surrounding what it means to care for our future self, or selves, at all.

Only one certainty: the induction of decision theory into the discussion makes Many Worlds debates sound, to the uninitiated, oddly psychological.

For better or worse, there’s no technically feasible experiment that can be done to adjudicate between a collapse hypothesis and an Ev­erettian noncollapse hypothesis. The literature provides only one even somewhat reasonable suggestion, “quantum suicide,” and even this offers no proof, only increasingly persuasive evidence. The quantum suicide experiment proposes that an ex­perimenter play a serial game of Rus­sian roulette—that urban myth whereby a person randomly loads one of the six chambers of a re­volver with a bullet, fires at herself, then respins the cylinder, then fires again, etc. If the experimenter plays the game again, and again, and again… and finds herself still alive, she should (if she’s logical), with each blank shot, find herself in­creasingly convinced by what Ev­erett proposed. Because although the first time she shot herself, she had an 83 percent chance of surviving, by the tenth shot she had only a 15 percent chance of surviving, by the twentieth a 2 percent chance, and the chances only go down from there. So if, despite the odds, after one thousand shots she’s still alive, well, only an Everettian ontology guarantees the reality of such an un­likely scenario of survival.

VII. The Problem of Missile-wielding Contemporaries

Despite courting death through the usual means of smoke and drink, Everett himself is not known to have pursued any such verification of his theory. The austerely mathematical nature of Everett’s thesis work, and his subsequent aloofness, make it difficult even to be certain whether all these elaborations on and defenses of his original idea are at all in line with what he might have intended; Everett himself moved on to other problems.

While in Copenhagen, after being brushed off by Bohr, Everett went to a bar, drank and drank, and then, on three sheets of hotel letter­head, made the early notes on what would become the Everett Algorithm. The idea behind the al­gorithm was to apply Lagrange multipliers—a method of finding the mathematical extremes of solutions to multivariable problems—more generally to more complicated problems. The Everett Al­gorithm was used on what are known as optimization problems. One classic example of an optimization problem is how best to lay out and time traffic lights; another is how to design a missile so as to maximize damage done.

Everett worked on military and civilian optimization problems both. Much of the military work remains classified, but it is known that Everett worked on the Mi­nute­man Missile Project, co­authored the paper “The Distribution and Effects of Fallout in Large Nuclear Weapon Campaigns,” and participated in UFO research. Everett explored the most efficient and sensible bussing system to reduce racial segregation. The leading war game models of the 1960s and 1970s came from Everett, specifically from his continued work applying Lagrange mul­tipliers to optimization problems. But what is game theory but the anal­ysis of all possible outcomes? In a sense, all of this research can be thought of as a kind of rigorous speculation about possible worlds; the only difference between Many Worlds thinking and this kind of thinking is how seriously you take the ontology, how insistent you are about the formalism corresponding to reality. The tradition of Possible Worlds in philosophy, which is distinct from Many Worlds, has often argued that the sort of if-then talk of counterfactual situations, the if-then talk implied by physical laws (if I drop this rock off the tower, then it will fall with this ve­locity…), only makes sense if you consider those other counter­factual worlds to actually exist. So Everett did abandon his Many Worlds work, but also he didn’t.

VIII. The Problem (and nonproblem) of Misappropriating Mystics

Perhaps the most pervasive and sloppy appropriation of scientific theory into popular rhetoric has been the “hey, it’s all relative!” take on Einstein’s special theory of relativity. Special relativity actually builds itself around an absolute, perspective-independent, categorical fact about the speed of light in empty space. And although special relativity proves the time distances between events perspective-dependent, it also proves the space-time distances wholly, utterly, eternally fixed. So special relativity could just as well have been called the special theory of absoluteness. One might wonder whether such a name might have precipitated (and possibly did in a paralleliverse?) an entirely different political and social discourse—although any such wondering seems to presuppose that proverbial hammers don’t see the whole world as proverbial nails.

From the beginning, the susceptibility of Everett’s work to misappropriations troubled Everett’s advisor, John Wheeler. In 1955, in response to Everett’s draft thesis, Wheeler wrote that he was “frankly bashful about showing it to Bohr in its present form” as it was “subject to mystical interpretations by too many unskilled readers.” Time has proven Wheeler’s worry well founded. Even without MWI, quantum mechanics has been ap­propriated into the popular culture in embarrassing, arguably even evil, ways: Deepak Chopra makes millions advocating “quantum healing” (his book of that title was published in 1989), an idea that skates wildly across quantum me­chanics’ vocabulary in order to convince people that, if they could just think positively, cancer couldn’t kill them. Similar ideas were pushed in the outrageously successful (especially financially) film What the Bleep Do We Know!? (2004), a kind of in­fomercial for the highly profitable “Ramtha’s School of En­lightenment,” which offers $1,600 seminars with its leader, JZ Knight, who channels (with a thick accent) the thirty-five-thousand-year-old spirit Ramtha. One of the main MWI skeptics, David Albert of Columbia University, gets quoted extensively in the film explaining quantum mechanics; Albert says his views in the film are “radically misrepresented.”

The Many Worlds Interpretation tends to find itself in related company, featured in the online writings of conspiracy theorists and armchair mystics. Everett wasn’t in­different to these possible perverse attentions; in a 1977 letter to a fellow physicist inquiring about Many Worlds, Everett emphasized that he hadn’t originated the Many Worlds moniker and furthermore that he “had washed my hands of the whole affair in 1956.” However, certain of Everett’s habits are inevitable magnets for kooky speculation. He always carried a micro­film camera. One of his main hobbies was CB transmitting; his CB buddies called him “The Mad Scientist.” And he particularly liked both ocean cruising and square dancing. Also: most of the work he did for the Pentagon remains labeled either “Secret” or “Top Secret.”

Regardless, even without the “suspicious” parts of Everett’s work and life, one could make an argument in favor of the kind of wild free-associating that happens when scientific terms make their pol­len-ous way into popular culture; after all, it’s generative. One might even suggest that, thought of in this light, the provenance of Everett’s idea really was science fiction; perhaps Everett brilliantly twisted the notion of alternate universes out of all recognition, and wrested it from its context, thus making it his own, but the seed—or sand—was nevertheless alien.

IX. Should a Cat Want to be Resurrected?

A  contrite and self-deluding believer in a Many Worlds type of ontology narrates Borges’s 1941 short story “The Garden of Forking Paths.” The narrator’s ancestor had written a confounding novel whose secret has been unlocked by a sinologist, Stephen Albert. Albert explains to the narrator, the novelist’s descendant:

In all fictions, each time a man meets diverse alternatives, he chooses one and eliminates the others; in the work of the virtually impossible-to-disentangle Ts’ui Pen, the character ­chooses—simultaneously—all of them. He creates, thereby, “several futures,” several times, which them­selves proliferate and fork… in Ts’ui Pen’s novel, all the outcomes in fact occur.

Shortly after the explanation, the narrator reluctantly murders Albert, for reasons at once martial, prideful, and random; but the narrator also entertains the absolving hope that Ts’ui Pen’s novel was visionary, that there really do exist other tracks of time in which the murder never occurred, in which he and Albert are, in fact, good friends.

This “positive” take on a Many Worlds ontology—those comforting other versions of our life coursing alongside us—comes to mind perhaps first. Mention Many Worlds to your friends and see how many of the responses fall into the category of, “So, like, in one of those other worlds we didn’t invade Iraq?” Or, “So in one of those other worlds, I’m the lucky seventh caller in an iPod giveaway?” But the disturbing logical inversion of such thinking lies in wait as well, for whoever likes to go looking for such things.

David Lewis, the late philosopher-father of modal realism, gave a lecture in June 2001 (“How Many Lives Has Schrödinger’s Cat?”) that speculated about Many Worlds’ implications of an eternal life. At the time, Lewis was suffering from late-stage diabetes; he lectured not long after receiving a kid­ney transplant, and just a few months—so it turned out—before he died. He began his talk by ex­plaining first superpositions, then both collapse and noncollapse in­terpretations of quantum mech­anics; finally he imagined what it might be like to be Schrödinger’s cat. If collapse dy­namics is true, each time an x-spin measurement is carried out—with an |up> measurement leading to poisoning and consequent death—the cat, upon being looked at, has a 50 percent chance of being alive. If the experiment is repeated, just as in the case of our Russian roulette player, the cat’s chances of survival continually diminish. However, if a non-collapse hypothesis, such as Ev­erett’s, is true, then the cat will always, no matter how many times the experiment is run, continue, in at least one paralleliverse, to live. Each run of the experiment as­suredly kills (one copy of) the cat, and assuredly does not kill ­(another copy of) the cat. Lewis calls this an “evil experiment.”

Lewis then points out how we are all Schrödinger’s cats. Even though we are not subjected to experiments, other kinds of superpositioned possibilities continually occur; every time one or another (or another) thing might happen to us, all of those things (if Everett was right) happen and don’t happen to us; some of those things can cause quite a bit of damage. “Chemical processes are no less ­quantum-me­chanical. These include biochemical processes… so such death-mechanisms as poisoning, in­fection, auto-immune disease, ven­tricular fibrillation, or heart fail­ure are also occasions for life-and-death branching.” The branches are simply terms of the superposition—but how pleasant are those life-branches? In an Ever­ettian world, all those possible branches (diabetes destroys your kid­ney and you die, diabetes de­stroys your kidney and you survive but are on dialysis, diabetes destroys your kidney but astonishingly you get along just fine without it) will actually exist. But in the majority of the possible branches for, say, a man suffering from late-stage diabetes, the man either dies, or suffers a further—but not quite fatal—deterioration.

Lewis goes on to engage the other “strangenesses” of quantum mechanics in his analysis of this larger-scale (our lives) “evil” quantum mechanical experiment. Be­cause of something called quantum tunneling, “If you stand in front of an oncoming bullet, there are branches (of stupendously low in­tensity, of course, and with neg­ligible chances of being the outcome of a collapse) in which the bullet passes right through you, leaving you unscathed, or less than fatally scathed. If you stand in front of an oncoming tram, there are branches of still lower intensity in which you reappear on the other side of the tram, or in which not all of you, but enough of you to sustain life, reappears.” In an Everettian noncollapse world, those branches of stupendously low intensity—in which some confettied version of you survived that on­coming tram—actually occur. In the vast majority of the other branches, you’re dead, but in the pre­ponderance of branches in which you do survive, you’re not feeling too good. And since any person, over time, will inevitably ac­crue dangerous encounters—with buses, with blood clots, with creepy microorganisms—survival tends to become less and less ap­pealing; imagine your standard fears of aging, and then multiply them times infinity.

Lewis admits that “to be sure, there are also life-and-life branchings such that on some branches your life is improved. Your previous losses are regained: your loved ones come back to life, or your eyes or your limbs grow back, or you regain your mental powers or your health…. but improvement branches have a very low share of total intensity… In the case of the worst dangers we face, the death branches have the most total intensity, the harm branches the next most, the status quo life branches have much less, and the improvement branches have by far the least.” The eternity an individual con­tinually steps into, never free of possible harms, therefore becomes increasingly hostile to survival, in­creasingly hellish (at least from the aging survivor’s point of view). “As you survive deadly danger over and over again, you should also expect to suffer repeated harms. You should expect to lose your loved ones, your eyes and limbs, your mental powers, and your health.” In the worlds in which you die, the reasoning goes, there’s no you there to enjoy the peace of that, and in the worlds in which you live, your life will almost certainly, in almost all branches, grow ever more miserable. Or, to put it in folksy terms, over time, one’s body inevitably becomes an in­creasingly unpleasant re­sidence for the soul. Lewis, the beloved bearded metaphysicist who had argued that the possible worlds of counterfactuals be considered to actually exist, concluded this final lecture of his life with:

Eternal life on such terms amounts to a life of eternal torment… You who bid good riddance to collapse laws, you quantum cosmologists, you enthusiasts of quantum computing, should shake in your shoes. Everett’s idea is elegant, but heaven forfend it should be true! Sad to say, a reason to wish it false is no reason to believe it false…. So, how many lives has Schrödinger’s cat?—If there are no collapses, life everlasting. But soon, life not at all worth living. That, and not the risk of sudden death, is the real reason to pity Schrödinger’s kitty.

But even the positive takes on Many Worlds can lead to unsettling outcomes. Not long after Everett’s death, his mentally ill daughter committed suicide; in the note she left behind she wrote that she was leaving to find her father in a parallel world. But, as Lewis says, whether or not we should want something to be true is entirely separate from whether or not it is true. And despite accumulating ar­guments against MWI, as philo­sopher and skeptic Sidney Felder notes, “Everett’s theory has many theoretical and technical ad­vantages, and this (together with des­peration about the pros­pects of more conventional ap­proaches) is why interest in this extravagant picture of the world continues to grow.”